One-Factor ANOVA (RM Design)
Your homework problem:
You are interested in the effects of arousal on motor performance. A random sample of subjects perform a complex motor task under 3 conditions: no caffeine (low arousal), a small dose of caffeine (moderate arousal), and a large dose of caffeine (high arousal). The dependent variable represents performance level: higher scores represent better performance.
This study resulted in the following data:
| Subject | Low Difficulty |
Moderate Difficulty |
High Difficulty |
| 1 | 15 | 17 | 19 |
| 2 | 2 | 7 | 4 |
| 3 | 11 | 12 | 6 |
| 4 | 13 | 15 | 5 |
| 5 | 12 | 12 | 7 |
| 6 | 2 | 18 | 11 |
| 7 | 8 | 12 | 5 |
| 8 | 9 | 14 | 2 |
Did the perceived level of caffeine significantly affect the participants’ performance (alpha = .05)? If your analysis reveals a significant overall effect, then make sure to explore all possible mean differences with a post-hoc analysis (same alpha).
If you would like some help with your hand-written work, click here.
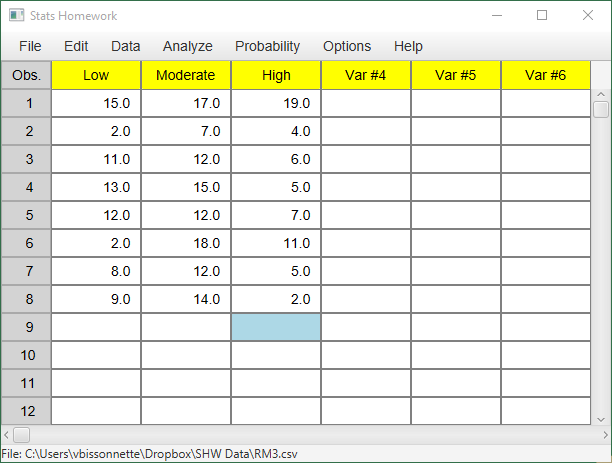
Enter these data into Stats Homework’s data manager and rename the variables. Your screen should look like this:
Make sure to double-check and save your data. To conduct your analysis, pull down the Analyze menu, choose Analysis of Variance, and then choose One-Factor ANOVA for Repeated-Measures Designs. You will be presented with this user dialog:
Move your three variables under “Dependent Variables,” select all the output options, and press the Compute button.
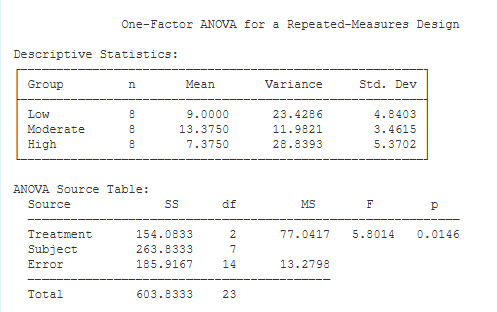
Basic Output
Descriptive Statistics. This table includes descriptive statistics for each treatment condition.
ANOVA Source Table. This table details the result of your analysis of variance (ANOVA). You have three sources of variance: treatment condition variance, subject variance, and error variance. Each variance component is associated with its own sum of squares (SS), degrees of freedom (df), and mean square (MS).
The F statistic is equal to MS(Treatment) / MS(Error) (5.80). Next to the F statistic is p — the chance probability / significance level of your result (.015).
Optional Outputs
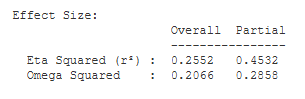 Effect Size. Eta Squared and Omega Squared describe the proportion of variance in your scores that can be attributed to your treatment effect. Omega Squared is an unbiased estimate of variance accounted for — i.e., it compensates for sample size.
Effect Size. Eta Squared and Omega Squared describe the proportion of variance in your scores that can be attributed to your treatment effect. Omega Squared is an unbiased estimate of variance accounted for — i.e., it compensates for sample size.
This table displays the overall effect sizes — these give you the proportion of the total variance accounted for. In addition, it displays the partial effect sizes — these give you the proportion of variance accounted for after you have removed the subject variance.
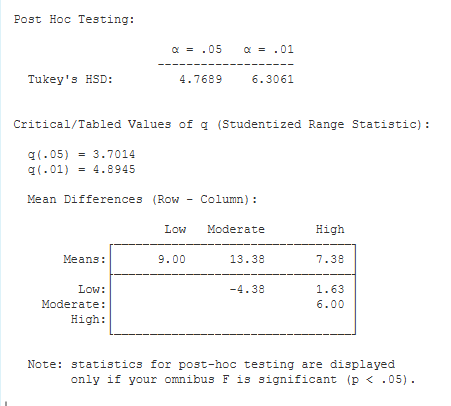 Post-Hoc Testing. If you request it, Stats Homework will also produce this output if your overall F statistic is significant. You are presented with the critical value of Tukey’s Honestly Significant Difference (HSD). Below this table is a table of mean differences. To conduct a post-hoc test, you would compare the critical value of HSD to each of the mean differences. If an obtained mean difference is greater than HSD, then you would conclude that this mean difference is significant.
Post-Hoc Testing. If you request it, Stats Homework will also produce this output if your overall F statistic is significant. You are presented with the critical value of Tukey’s Honestly Significant Difference (HSD). Below this table is a table of mean differences. To conduct a post-hoc test, you would compare the critical value of HSD to each of the mean differences. If an obtained mean difference is greater than HSD, then you would conclude that this mean difference is significant.
Also included in this output is the critical values of the Studentized Range Statistic (q), which is used to compute HSD.
Critical Values. These are the values from a statistical table of critical values for the F test. In our case, we are conducting a test with alpha = .05. So, we would compare the value of our obtained F (7.82) to 3.74.
Supplemental Statistics Used in Hand Calculations. These are statistics that can be helpful if you would like to double check your hand-written computations.
Box Plots. Finally, you will be presented with graphical box plots of your data. You can modify these plots in a variety of ways, save them to disk, or copy them to your clipboard.